Expert Tip: Concrete shear design based on AASHTO LRFD 1

Author: Seungwoo Lee, Ph.D., P.E., S.E.
Publish Date: 22 May, 2023
Concrete Shear Equation
The concrete shear equation is
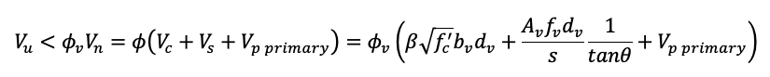
Traditionally AASHTO and ACI gave us β=2 and θ=45° and these values are also allowed at the current AASHTO LRFD for some limited cases as defined in 5.7.3.4.1.
Our fore-engineers were aware of these values are very rough estimates but had to wait until the mid-80s before professors at the University of Toronto fully developed the modified compression field theory through extensive theoretical and experimental research (Prestressed concrete structures by Collins and Mitchell, 1991).
Basically, this modified compression field theory requires some iterations, but with computers, there is no problem to perform this iteration. One of the most comprehensive programs using this method is Response 2000 which can be downloaded from Dr. Bentz’s website https://www.hadrianworks.com/ free of charge.
AASHTO LRFD 2nd edition (not sure about the 1st edition because the author does not have a copy) adopted this method and gave us design tables to find θ and β for given values of longitudinal strain at mid-depth of the member εx and vu/f’c, where vu is shear stress and f’c is the compressive strength of concrete. vu is defined as

From AASHTO LRFD 5th edition, these tables were moved into the appendix and AASHTO LRFD allows to use of a more simplified formula.

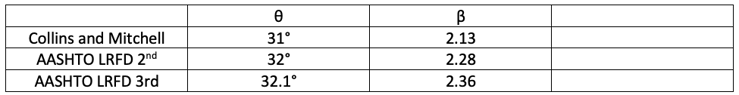
In this article, the design table, now in the AASHTO LRFD appendix will be discussed. Now three design tables are available, and all these give close values of θ and β.
Values of θ and β, psi unit, for members with web reinforcement
(Only shown for εx=0.5/1000, vu/f’c=0.15)
The Calculation Steps
The calculation steps are as follows:
Step 1) Assume θ=32°
Step 2)
Step 3) Assume ε1=0.003075
Step 4)
Step 5)
Otherwise, increase the section and repeat Steps 1) and 5)
Step 6)
Step 7)
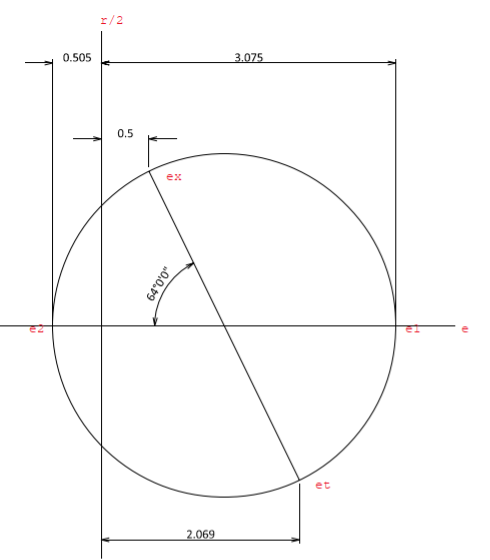
If εt<0.0002, reduce θ and repeat Step 1) to Step 8). Now we can draw Mohr’s circle.
Step 9)
Step 10)
Step 11)
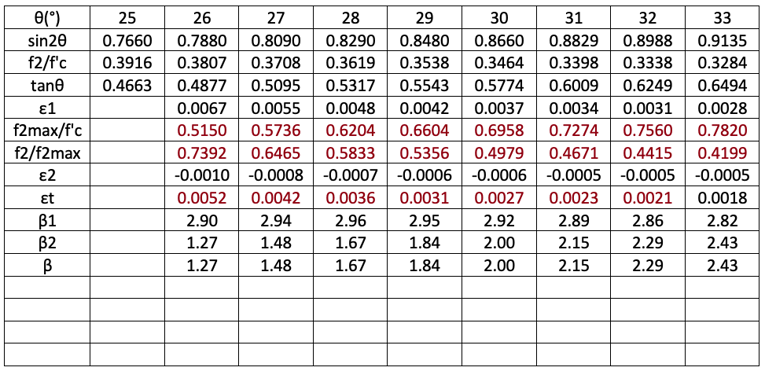
The same calculation can be performed for other values of θs.
If θ is less than somewhere between 25°~26°, f2 is larger than f2 max and no solution exists.
If θ is larger than somewhere between 32°~33°, εt<0.002, and the stirrup would not yield.
The valid range of θ is between 25°~33°.
With computers, we can easily calculate the valid range of θ as 25.2324°~32.2816° but has little meaning considering the uncertainty of concrete itself.
β max =2.29 with θ=32°, (or β=2.33 with θ=32.2816°) which is close to what the tables give.
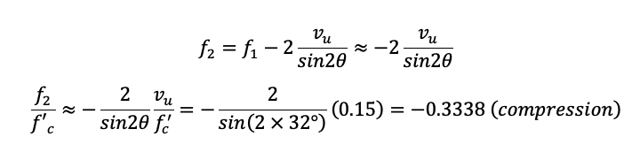
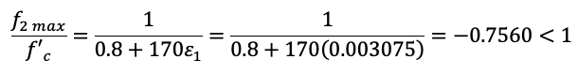
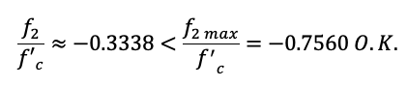

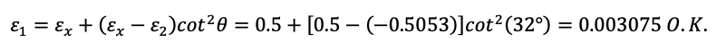
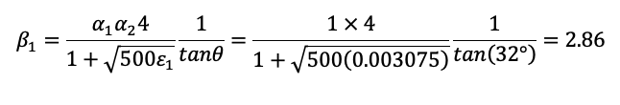
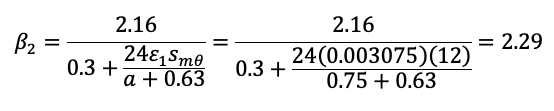
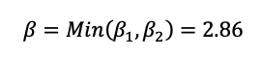



Add a Comment