Midas NFX: Thermal Analysis [Infograph]

Author: Gabriel Roade
Publish Date: 27 Dec, 2021

Learn about other kinds of analysis in our Finite Element Analysis Ultimate Cheat Sheet!
THERMAL ANALYSIS
THE STUDY OF THE PROPERTIES OF MATERIALS AS THEY CHANGE WITH TEMPERATURE
IN ENGINEERING, IT OFTEN REFERS TO THE STUDY OF HEAT TRANSFER AND THERMAL STRESS IN A STRUCTURE
Heat Transfer Mechanisms
Radiation
The exchange of energy in the form of electromagnetic waves.
Radiation needs no physical medium to travel through.
Radiation emanates from the surface of every object; any body of mass is a heat source.
The heat flux due to radiation from the surface of an object into the air surrounding it can be defined through the following equation, based on Stefan-Boltzman Law.

q = Heat Flux ; σ = Stefan-Boltzman Constant ; F = Radiation View Factor
ε = Emissivity of the object ; α = Absorptivity of surrounding air
T = Temperature of the object ; Tα = Temperature of surrounding air
Conduction
The phenomenon wherein heat energy travels between neighboring molecules due to a temperature gradient within the boundaries of an object.
If there is a temperature difference (gradient) in the object conduction will always occur, regardless of the state of the object (gas, liquid, solid).
Heat energy flows from parts of the object at a higher temperature to parts at a lower temperature.
The heat flux, defined as the rate of heat energy transfer through a unit area per unit time, due to conduction within an object is determined by Fourier's Law:

q = Heat Flux ; k = Thermal Conductivity of the material ; ∇T = Temperature Gradient
Convection
The phenomenon of heat transfer due to the movement of molecules within a fluid, either gas or liquid.
Convection most commonly originates from the boundary of a solid object surrounded by a fluid. For example, when a hot object slowly cools down when placed in air.
Depending on the cause of the flow of molecules, convection is categorized as free convection or forced convection.
Forced convection refers to the flow of a fluid due to external forces, such as the wind, a fan, or cooling water; moving a solid inside a fluid can also be considered as forced convection. Computational Fluid Dynamics (CFD) analysis is preferred rather than pure heat transfer analysis, so as to account for the mass flow of the fluid.
Free convection, or natural convection, refers to the flow of a fluid due to changes in the fluid's density caused purely by temperature differences. No external means are involved in free convection.
The heat flux due to convection at the boundaries between a solid and a fluid can be accurately approximated by Newton's Law of Cooling when the heat transfer coefficient is relatively independent from the temperature difference:

q = Heat Flux ; h = Heat Transfer Coefficient ; T = Temperature of the object
T0 = Temperature of the surrounding fluid
Heat Transfer Analysis
ANALYZING THE FLOW OF HEAT ACCORDING TO TEMPERATURE DIFFERENCES, AND THE RESULTING TEMPERATURE DISTRIBUTION AND TEMPERATURE CHANGES
Analyses are categorized according to heat transfer's...
Time Dependency
-
STEADY-STATE HEAT TRANSFER
A steady state happens when an object and the surrounding environment are at the same temperature.
In this state, the cause and speed of heat transfer and the temperature distribution are uniform and do not change according to time.
Steady-state heat transfer involves the analysis of the heat flow and temperature distribution in this steady state.
-
TRANSIENT HEAT TRANSFER
If heat transfer occurs, the cause and speed of heat transfer and the temperature distribution continue to change over time until a steady state is restored.
This intermediate state of change is referred to as a transient state.
Transient heat transfer involves the analysis of how the heat flow and temperature distribution change over time in this transient state.
Temperature Dependency
-
LINEAR HEAT TRANSFER
In this type of heat transfer analysis, the cause of heat flow and the material characteristics of the object do not change depending on temperature and stay constant.
Most heat transfer analyses that involve common materials, conduction, convection and heat flux conditions are linear heat transfer analyses.
-
NONLINEAR HEAT TRANSFER
Contrary from linear heat transfer, the cause of heat flow and/or the material characteristics of the object do change depending on temperature, which is the degree of freedom of heat transfer analysis.
Heat transfer analyses involving temperature-dependent material models or radiation heat transfer fall in this category.
Thermal Stress Analysis
EXAMINING THE SAFETY OF A DESIGNED PRODUCT IN RESPONSE TO THERMAL DEFORMATION CAUSED BY CHANGES IN TEMPERATURE DUE TO VARIOUS HEAT SOURCES
- Changes in temperature thermally impact the material.
- Most impacts involve thermal stress and/or thermal deformation, which alters the vibration of molecules inside the material.
- The vibration of molecules inside the material is directly proportional to the heat energy of the object.
- A decrease in vibrations stiffens molecular bonds, causing the material to contract.An increase in vibrations relaxes molecular bonds, causing the material to expand.
Thermal Deformation
The phenomena of expansion and contraction due to temperature changes can be expressed with the following equation:
εT = Thermal Strain ; ΔT = Temperature Change ; α = Thermal Expansion Coefficient
Thermal Expansion Coefficient
The rate of deformation per unit temperature. The following equation uses it to predict the amount of deformation due to heat:
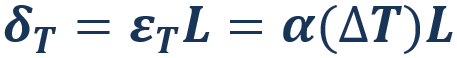
εT = Thermal Strain ; ΔT = Temperature Change ; α = Thermal Expansion Coefficient
δT = Thermal Deformation ; L = Length
Thermal Stress
Thermal stress caused by thermal deformation is sensitive to constraints.- If there are no constraints on an object expanding or contracting due to temperature changes, no internal force occurs in the object.
- If and object expanding or contracting due to temperature changes is constrained, internal forces occur, resulting in thermal stress.
σT = Thermal Stress ; εT = Thermal Strain ; E = Modulus of Elasticity
Thermal Analysis Steps
-
Heat Transfer Analysis
- Temperature Load
- Heat Transfer Analysis
- Temperature Distribution
- Automatic conversion of Temperature Distribution to Thermal Load
-
Thermal Stress Analysis
- Thermal Load
- Thermal Stress Analysis
- Deformation
Conduct your analysis for FREE!

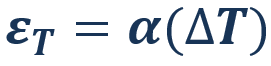


Add a Comment